Economics Terms A-Z
Savings-Investment Identity
Read a summary or generate practice questions using the INOMICS AI tool
The savings-investment identity in economics is a macroeconomic concept related to aggregate demand, the IS Curve and the market for loanable funds. This identity states that the amount of funds available for investment activities must be equal to the amount of money saved in the economy. It can also be thought of as an accounting identity, as it is true by definition.
It’s helpful to define the term “investment” in an economics sense before continuing. The word “investment” in finance and in everyday discourse usually refers to the buying and selling of assets like financial securities. However, in economics, investments are economic activities that have potential to grow the economy. They can be conducted by private firms, governments or other entities (like entrepreneurs) Examples include the building of a new university, the construction of a manufacturing facility, or the R&D expenses used to develop a new medication.
When firms or other economic agents want to make an economic investment, they must typically take out a loan, usually with the help of a bank to act as an intermediary. The money comes from funds that economic agents have saved, which are then considered loanable funds since these are the funds banks can repackage and make available for borrowing as loans.
So, the savings-investment identity holds true by necessity. The amount of money available for borrowing is equal to the amount of savings that economic agents have stored, because those savings are converted into loanable funds.
Describing the identity mathematically
We can show this identity mathematically by using the formula for GDP and making some assumptions. The relevant formula is: GDP = C + I + G + NX, where C stands for consumption, I stands for investment (defined just as we’ve discussed above), G is government spending, and NX represents net exports.
As a side note, readers may notice that this is the same as the equation for aggregate demand (AD), and that’s because GDP is one measure of Y, or economic output. When the economy is in long-term macroeconomic equilibrium, Y as measured by GDP is equal to AD and both short-run and long-run aggregate supply.
Returning to the equation, let’s assume that the economy under consideration does not trade with any others, so that the NX term is zero. Let’s also assume that aggregate demand and both short-run and long-run aggregate supply are in a stable long-term equilibrium, which means that GDP = AD = SRAS = LRAS = Y. These two assumptions allow us to replace GDP with economic output Y and ignore NX, transforming our equation to: Y = C + I + G.
Now subtract the portions of GDP that account for spending activity, yielding: Y - C - G = I. This shows that economic output minus consumption and government spending is equal to investment. We can replace the term on the left-hand side with one “new” term: savings S. This gives us the savings-investment identity S = I. Macroeconomics courses may define Y - C - G = S straight away, but let’s walk through the logic behind this idea further.
To see how S = Y - C - G, consider the following. When rational economic agents have money on hand, they must choose how to allocate that money among two basic options: spending it or saving it. Every use of money falls into one of these two basic categories.
Money that is not spent must be saved, and vice versa, or else money is being irrationally lost or is going unused. While that is possible in the real world, it’s common and useful to assume that all money not used for consumption is put into savings, such that there is no money lost or “wasted”. (Even quite advanced economics courses typically assume this, since wasting money represents a Pareto inefficiency, and is an irrational “decision” facing no meaningful tradeoffs, making it economically irrelevant. It is always economically optimal to not waste money.)
Considered from an economy-wide perspective, then, economic agents do not waste money and must choose between two basic options: save or spend the money they have. The total amount of money they have is Y (remember that GDP can be considered the sum of all income in the economy!).
Then, the government, individuals (or households) and firms choose to spend some of their money on goods and services, increasing the spending categories C and G as they do. In this example, all spending falls into either C or G. Finally, any money that governments, firms or consumers have left over becomes savings, S, which is used in the loanable funds market to supply borrowers with loans. Then, these borrowers use those loans to invest in economic investment projects that will grow the economy. Thus, as long as money isn’t wasted or destroyed, S = I, and Y = C + I (or S) + G. See the diagram below for a depiction of this process.
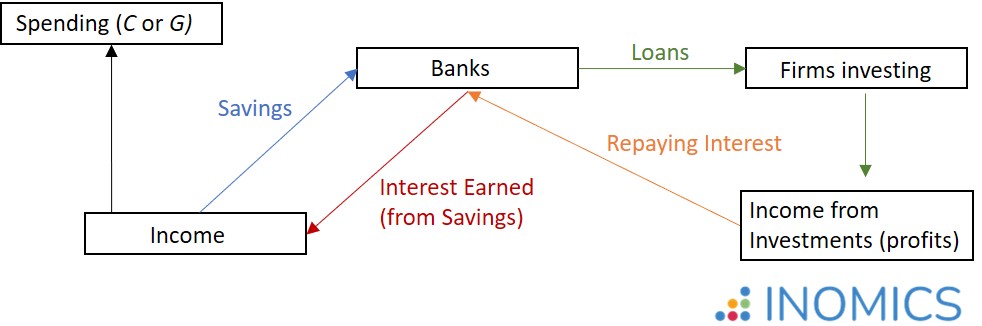
Incidentally, the proportion that consumers choose to save – which can change depending on people’s preferences and the state of the economy – is determined by the marginal propensity to consume. Readers interested in this effect should read the linked article.
A note on taxes
Taxes have been hidden in our analysis thus far, but it helps to include them formally. Before, we did not do so because in a closed economy without trade, all government spending G must be funded by taxes T taken from domestic consumers. So, in the previous section, G = T and Y is not affected by the level of taxes, since all taxes taken from consumers’ incomes (C and I) are used in G as the government’s income (of course, this assumes there is no deadweight loss from taxation).
Another way to view the previous section’s equation, then, is: total national income Y equals consumption spending C plus savings S (aka investment) plus taxes T; Y = C + S + T. In other words, the total income for consumers Y is spent on either consumption, savings, or taxes. This corresponds to the income method of constructing GDP discussed in the linked GDP article.
Now with trade: the identity in open vs. closed economies
In this section, we consider “open economies” to be those that trade with other countries, while “closed economies” are those that are not open to any trade (they are autarkic). The above discussion is accurate for closed economies.
The savings-investment identity works similarly as before for open economies, with one key difference: in open economies, foreign economic agents can invest in the domestic economy, and domestic agents can save in foreign economies. If the balance of these financial flows is not equal to zero, the NX term reappears in our equation: Y = C + I + G + NX.
We can further break up NX into two terms, imports M and exports X, so that net exports NX = X – M. Now it’s clear to see that NX is negative when there are more imports than exports, decreasing Y. This means domestic agents are spending more money to acquire foreign goods (imports) than foreign agents are spending to buy domestic goods (exports). This lowers Y, and ceteris paribus means that there is less investment in the domestic economy. Instead, domestic agents are sending money abroad (by purchasing foreign goods).
Conversely, if exports X are greater than imports M, NX is positive. This is the case when domestic agents are spending less abroad than foreign agents spend domestically. This grants the domestic economy more savings than it would have otherwise had, potentially increasing the amount of investment than would be possible with only domestic agents saving.
Incidentally, the balance of imports and exports may be referred to as a trade balance. This also affects an economy’s capital accumulation. When X > M, it is said to be a trade surplus and it causes a “net capital inflow”, and when M < X it is a trade deficit and causes a “net capital outflow”.
Mathematically, we must modify the closed economy version of the formula to account for these new possibilities. Now, our full equation is Y = C + I + G + X - M. Notice that if X = M (the case with an even trade balance), our analysis is exactly the same as before. And finally, before proceeding, note that S is now the total amount of savings in the domestic economy, from all sources.
We have two equations for Y; the “aggregate demand” version just defined and the income equation Y = C + S + T. Setting these two equations equal yields C + S + T = C + I + G + X - M. Consumption spending cancels to yield: S + T = I + G + X - M. Subtract taxes to finally yield the savings equation in this open economy: S = I + G - T + X - M. Now, savings in the domestic economy equals savings by domestic agents I, plus government saving G - T, plus savings by foreign agents X - M. It’s also clear that if government income and spending are equal, and if the trade balance is perfectly even, savings equals investment as before.
Readers may be confused by the above. How can the identity still hold, if the balance of trade isn’t even? To see this, recall that S in the above equation is total savings in the domestic economy. It’s possible for total savings in an open economy to be greater than the amount saved by domestic agents in a closed economy, if foreign agents save money in the domestic economy. This may seem like a violation of the identity. But, it isn’t, because foreign agents saving domestically represents income or investment for a domestic agent to utilize, growing the economy more than it could have on its own.
In this article, we’ve assumed that foreign money only enters the domestic economy when foreign agents purchase domestic goods and services, increasing X. Yet in the real world, it’s possible (and quite common) to outright purchase foreign assets or save money in foreign economies. This doesn’t change our analysis, however; these activities can simply be considered a part of exports in our simplified model.
Finally, note that the savings-investment identity must hold globally as well. If all savings and all investments in the world are summed, they will be equal. To see this, redefine the “domestic” economy as the entire world. In this case, there is no foreign trade, only trade among domestic agents. Then, the equation simplifies to the closed economy case above where I = S = Y - C - G.
Further Reading
The IS Curve is a concept that follows directly from this description of the savings-investment identity and the goods market. It describes in a single curve the equilibrium points for the goods market where investment equals savings, and is used as one of the main portions of the IS-LM model in macroeconomics.
The liquidity preference model and the demand for money form the LM part of the IS-LM model.
-
- Professor Job
- (Hybrid)
- Posted 1 week ago
Visiting Professor in Economics, Management and Statistics
in Lucca, Italie
-
- Professor Job
- Posted 1 week ago
Tenure-track Faculty Position in Economics
At Kutztown University of Pennsylvania in Kutztown, États-Unis
-
- Professor Job
- Posted 1 week ago
Associate Professorship(s) in Business Economics
At Aarhus University in Herning, Danemark













