Nash equilibrium is an important equilibrium or solution concept in non-cooperative game theory. A Nash equilibrium is a strategy profile (i.e. a strategy for each player) in which each player is playing the best response to the strategy of the other(s).

Many games in life have a Nash equilibrium of some type, and learning the Nash equilibrium can help economists predict human behavior (at least, when people behave rationally and selfishly in an economic sense). For example, the foundational Prisoner’s Dilemma features one Nash equilibrium. Other games may not feature one, however, particularly games with an unlimited set of possible choices.

Put simply, a Nash equilibrium describes a situation in which each person acts optimally given the actions of the others, so that no one wants to change his or her action. The concept is named after the American mathematician John Nash (1928-2005) who formally developed the concept in the 1950s and won a Nobel Prize in Economic Sciences for his work.

In many situations we are confronted with different options and have to decide which one to choose. The payoff (or utility) that we obtain from each option depends both on our own decision and on what others decide.

For example, if I can commute to work either by car or by metro, how comfortable the car ride is depends on traffic. Meaning, how many others decide to commute by car affects my utility. A situation is a game when two or more decision makers (or players) decide between two or more options, and the players' decisions also affect each other’s payoffs.

In a game, each player takes into account the immediate effect of his or her decision on his or her own payoffs and how the other players will react. As we assume that players are rational and their objective is to maximize their own payoff, we know that every player will play a "best response" to the strategy of the others. 

An action is the best response if it gives the player a higher payoff (or at least the same payoff) than the payoff that he or she can get by choosing any other possible action.

The (Nash) equilibrium of a game

The Nash equilibrium of the game corresponds to a situation where no one has an incentive to change his or her strategy given the strategies of the others ‒ i.e., when everyone is playing their best response. This implies that in a Nash equilibrium, the actions that people take coincide with the actions that the others think they will take.

Given that everyone correctly anticipated what the others would do and everyone chose the best alternative considering the strategies of the others, no one can gain by deviating from their current strategy. In other words, everyone is happy with the decision that they took in view of the others' decisions.   

An example (coordination game)

Let's consider the concept of Nash equilibrium with the help of an example. Suppose there are two friends, Alex and Blake, who decide to meet in the park. Both of them enjoy playing basketball, but neither of them wants to be the one to bring the ball from home to the park. Each of them can choose between two options: either bring a ball from home or not bring a ball.

The payoffs in this game could be the following: if no one brings a ball, everyone receives a payoff of zero. If both bring a ball, then everyone gets a payoff of 1. If only one brings a ball, the person who brings the ball gets a payoff of 1, while the other one gets a payoff of 2.

We can write down the actions and payoffs in form of a payoff matrix:

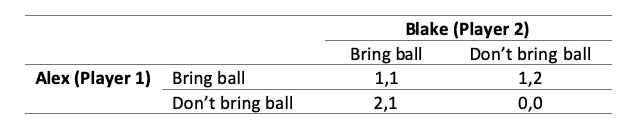
In each of the four cells displaying the payoffs, the number before the comma corresponds to the payoff of player 1, in our case Alex. The second number describes the payoff of Blake. This means that if both Alex and Blake bring a ball everyone gets a payoff of 1, and so forth.

Now let us find the Nash equilibria of this game. To do so, we check what is the best response of Alex for any action of Blake. If Blake brings a ball then Alex gets a payoff of 1 if he also brings a ball and a payoff of 2 if he does not.

Consequently, his best response to Blake bringing the ball is not to bring a ball. If Blake does not bring a ball, Alex obtains a payoff of 1 if he brings one and a payoff of zero if he does not. Hence, his best response to Blake not bringing a ball is to bring a ball.

Now we can repeat this exercise to find the best responses of Blake and we see that she should bring a ball if Alex does not and should not bring one if Alex does. This means that this game has two Nash equilibria (in pure strategies). In the first Nash equilibrium, Alex brings the ball. In the second one, Blake brings the ball.

Why are these action profiles Nash equilibria? Because when Blake does not bring a ball and Alex does, neither is incentivized to change their decision given the decision of the other. That is, because Blake does not bring the ball, Alex brings it himself.

Why is both of them bringing a ball not a Nash equilibrium? Because if Alex knows that Blake is bringing a ball, it's not in his best interest to bring one himself. This means that in this situation he can gain (increase his payoff) by changing his strategy.

Mixed-strategy Nash equilibrium

The game described above is called a coordination game and has two pure Nash equilibria. A mixed strategy equilibrium is a Nash equilibrium in which one or more players randomize. This means that they play each strategy with some probability.

In symmetric games (as the one described above) where each player has the same possible actions and the same payoffs associated with each action, the focus is often to find the symmetric mixed-strategy equilibrium. In a symmetric mixed-strategy Nash equilibrium each player assigns the same probability to a particular action.

For the symmetric mixed-strategy Nash equilibrium of the game described above, each player assigns a probability of ½ to each strategy. Put differently, if each of them tosses a coin before leaving and takes a ball if the coin shows heads and leaves the ball at home if the coin shows tails, this strategy would be a (symmetric mixed-strategy) Nash equilibrium.

Note that in this equilibrium it is possible that they will have 2 balls and it is possible that they will have no ball at all depending on the outcome of the coin tosses. Yet, if Alex knows that Blake will toss a coin to decide what to do, his best response is to toss a coin as well. 

Further reading

Nash equilibrium is not the only solution concept for non-cooperative games. Depending on the information that the players have, how often they interact (i.e. how many time periods) or whether the players choose their actions simultaneously or sequentially, different solution concepts exist. Many of these solution concepts are refinements of the Nash equilibrium as e.g., the Subgame Perfect (Nash) equilibrium or the Bayesian Nash equilibrium (see e.g. “Game Theory: An Introduction” by Steven Tadelis, 2013).

Good to know

The movie “A beautiful mind” is based on the life of the American mathematician John Nash, who suffered from schizophrenia. His dissertation on non-cooperative games for which he obtained his PhD from the University of Princeton was only 20 pages long.